Linking maths to the real-world

Introduction

In high school and at pre-university levels, most maths lessons focus on the abstract, which can turn learners off and lead them to believe that  mathematics is not relevant to their daily lives. Since the beginning of the 21^st century, educational administrators and teachers have been focusing on how mathematics can be applied in the real-world. According to Vassiliou (2011), since 2007, most European countries have focused on improving the mathematical skills of students in solving real-world problems, rather than theoretical and abstract mathematical concepts. Most students prefer to solve maths problems when they can see how their learning can be applied in real life, so considering appropriate practical problems for use in the classroom plays an important role in increasing students’ interest (Vos, 2018).

In educational research, any real-world problem that is solved using mathematics is called a ‘practical problem’ (Gholami & Sathar, 2021; Savizi, 2006). In maths lessons, the ‘practical problem’ refers to the potential application of this mathematical knowledge in the real-world.

In the building frontage industry, civil engineers usually use their knowledge and experience to shape composite sheets to cover the convex and concave parts of buildings. The curved composite sheets are usually attached next to each other like a puzzle in order to cover the building’s frontage. However, when using this traditional method, some curved composite sheets are unusable due to the level of curvature. Therefore, not only are the costs and engineer’s workloads higher, but the quality of work suffers because of the presence of waves on the finished work surface. The convex (concave) part of a building is an arc structure of a circle. If engineers have the radius of this circle, they can use an advanced engineering machine to curve composite sheets to the exact curvature required.

The process of finding the radius of this circle was presented as a practical problem to students as a way for them to learn about the concept of the circle equation in a more interesting way. In fact, finding the radius of a circle requires using certain data about it. This practical problem in the topic of circle equation was designed as a lesson for use in mathematics classes. This course is not limited to a specific area, and mathematics teachers in all countries of the world can use it in their classes. Therefore, the purpose of this article is to introduce a new method in teaching mathematics and highlight the role of practical problems in teaching mathematics.

Circle equation

A circle is defined as the locus of a point which moves in a plane such that its distance from a fixed point in that plane is always constant. The equation of a circle with the centre (h,k) and the radius r is as follows:
〖(x-h)〗^2+〖(y-k)〗^2=r^2.
It seems that by using practical problems which require understanding of the circle equation help students to better learn this concept (Tong et al, 2020). Therefore, after discussing the theory behind the circle equation in the class, the teacher then introduces this practical problem to students and asks them to solve it using the circle equation and some basic mathematical concepts. If we know the coordinates of at least three points on a circle graph then we can find the equation of the circle through several different solutions.

A practical problem for frontage of the convex part of a building

Figure 1 represents a building with convex structure; the frontage of this part with composite sheets based on experience faces difficulties such as more costs and less quality. In this practical problem, an arc of a circle is given and engineers need having the radius of this circle in order to curve the composite sheets exactly similar the convex part of the building by using an advanced machine. Therefore, the researcher translated this real-world problem to a practical problem to prepare a mathematics lesson for students and ask them to find a formula for solution of this practical problem. So, considering this practical problem in the classroom provides an opportunity for learners to discuss about an application of the circle equation in the real-world.

Description of the practical problem

The researcher considered a mathematical graph for this practical problem as shown by Figure 2.

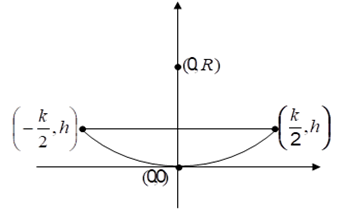
Based on the above figure, the two values h and k are given, and so we have the following data about a circle. The point M(0,R) is the centre of the circle and three points, N(-k/2,h), E(0,0) and F(k/2,h) which are located on the graph of this circle. We need to find the value for the radius.
Although there are a lot of ways to solve this practical problem, the researcher discussed two solution methods for it in order to students learn some properties of the circle equation.

These solution methods are explained as follows:

1: First method of solution
Based on Figure 2, the coordinates of three points N, E and F satisfy in the circle equation:
〖(x-α)〗^2+〖(y-β)〗^2=R^2.
So, we can obtain the three equations as the following (in this research, the symbol C(M,R) used for a circle with the centre M and the radius R).
N∈C(M,R)⇒(-k/2-α)^2+(h-β)^2=R^2⇒k^2/4+α^2+αk+h^2+β^2-2hβ=R^2
E∈C(M,R) 〖⇒(0-α)〗^2+(0-β)^2=R^2⇒α^2+β^2=R^2
F∈C(M,R)⇒(k/2-α)^2+(h-β)^2=R^2⇒k^2/4+α^2-kα+h^2+β^2-2hβ=R^2
Therefore, we have:
{█(k^2/4+α^2+αk+h^2+β^2-2hβ=R^2@k^2/4+α^2-kα+h^2+β^2-2hβ=R^2 )┤
Since α^2+β^2=R^2,
{█(k^2/4+αk+h^2-2βh=0@k^2/4-αk+h^2-2βh=0)⇒┤ {█(k^2+4αk+4h^2-8βh=0@k^2-4αk+4h^2-8βh=0)┤
⇒2k^2+8h^2-16βh=0
β=R⇒2k^2+8h^2-16Rh=0⇒R=(k^2+4h^2)/8h.
Therefore, we can find the radius of the circle by using the following mathematical formula:
R=(k^2+4h^2)/8h.

2: Second method of solution
Theorem: When two chords intersect each other inside a circle, the products of their segments are equal.
According to Figure 2, the two vertical and horizontal chords of the complete circle intersecting each other in the point (0,h). So, as respect to the above theorem, we have the following equation,
k/2×k/2=h(2R-h)⇒k^2=4h(2R-h)⇒8Rh=k^2+4h^2.
Therefore, we have R=(k^2+4h^2)/8h.

Discussion and Conclusion

Using practical problems and applications of mathematics in the real-world helps students to believe that mathematics is an applicable science in their daily life. This form of practical problem solving is far more interesting for students than abstract theoretical problem solving. Therefore, teachers can increase the interest of students in learning mathematics through considering some practical problems in their lessons.

This study shows an application of circle equation in the real-world about the frontage of convex (concave) part of buildings. Based on the mathematical formula in this research, engineers can curve the composite sheets like the convex (concave) parts of a building by using an advanced machine. So, the quality of building frontage improves significantly. Considering this practical problem in teaching helps students to see an application of the circle equation in the real-world. Therefore, they learn many new properties about the circle equation in a more engaging way. This teaching method not only helps students to experience the beauties of mathematics, but also shows the importance of mathematics in the real-world.

References
Gholami, H, & Sathar, M H A, (2021) THE APPLICATION OF CIRCLE EQUATION IN BUILDING COMPOSITE FRONTAGE. Advances in Mathematics: Scientific Journal, 10(1), 29–35.
Savizi, B, (2006) Applicable problems in the history of mathematics: Practical examples for the classroom. Teaching Mathematics And Its Applications, 1–6.
Tong, D H, Loc, N P, Uyen, B P, & Cuong, P ,. (2020). Applying Experiential Learning to Teaching the Equation of a Circle: A Case Study. European Journal of Educational Research, 9(1), 239–255. doi.org/10.12973/eu-jer.9.1.239
Vassiliou, A. (2011). Mathematics in Europe: Common challenges and national policies. Education, Audiovisual and Culture Executive Agency. doi.org/doi:10.2797/72660
Vos, P. (2018). “How Real People Really Need Mathematics in the Real World” Authenticity in Mathematics Education. Education Sciences, 8, 1–14.